Hadrons are emergent phenomena of QCD at distance scales greater than about 1 fm, where the dynamics is governed by the spontaneous breaking of chiral symmetry, color confinement, and topological effects. Explaining hadron structure and interactions requires methods based on effective degrees of freedom and clear principles for constructing the effective dynamics. Chiral Effective Field Theory (χEFT) expresses the dynamics of pions emerging from chiral symmetry breaking and permits a systematic description of low-energy pion and nucleon interactions and long-distance hadron structure. The large-Nc limit of QCD (limit of large number of colors) transforms QCD into an effective theory of hadrons with semiclassical dynamics and enables a systematic classification of meson and baryon structure and interactions. Complex analyticity (dispersion relations, sum rules) connects the behavior of hadronic amplitudes at low and high energies and relates the amplitudes of different processes. These methods are systematic (parametric expansions, exact relations) and describe hadron structure with clear guiding principles and controlled accuracy (uncertainty estimates). They provide both quantitative predictions and qualitative insights into the organization of the large-distance dynamics emerging from QCD. They are synergistic with Lattice QCD and can be used to explain lattice and/or accept lattice data as input.
The Theory Center is pursuing a focused research program using methods such as Chiral EFT, large-Nc QCD, and others, to explain large-distance hadron structure and interactions, particularly structures measured in electromagnetic scattering processes at JLab 12 GeV and other facilities.
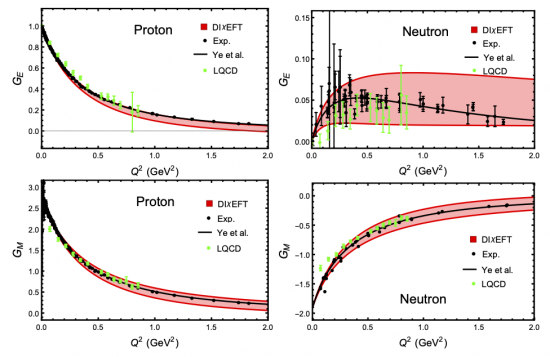
Nucleon form factors obtained from a theoretical calculation combining χEFT and dispersion theory (DIχEFT): red bands DIχEFT predictions [1], including the uncertainty range. Green points: lattice QCD results [2]. Black line: empirical form factor parametrization [3]. Black points: data points of Mainz A1 experiment [4,5].
Applications include
- Nucleon EM form factors and proton radius extraction, using χEFT and dispersion relations
- Low-energy Compton scattering on nucleon, using combined χEFT and 1/Nc expansion
- Spin effects in low-energy e-N scattering, using combined χEFT and 1/Nc expansion
- Transverse charge/magnetization densities, energy-momentum tensor, and nucleon generalized parton distributions (GPDs) using χEFT
- Partonic structure of nucleon (sea quark parton distribution functions (PDFs)) using local and nonlocal χEFT
- Strange baryon mass and EM properties, using 1/Nc expansion and lattice QCD data
- Nucleon scalar form factor and sigma term, using χEFT, 1/Nc expansion, and dispersion relations
[1] J. M. Alarcón and C. Weiss, Phys. Lett. B 784, 373 (2018).
[2] C. Alexandrou, M. Constantinou, K. Hadjiyiannakou, K. Jansen, C. Kallidonis, G. Koutsou and A. Vaquero Aviles-Casco, Phys. Rev. D 96, 034503 (2017).
[3] Z. Ye, J. Arrington, R. J. Hill and G. Lee, Phys. Lett. B 777, 8 (2018).
[4] J. C. Bernauer, et al. [A1 collaboration], Phys. Rev. Lett. 105, 242001 (2010).
[5] J. C. Bernauer, et al. [A1 collaboration], Phys. Rev. C 90, 015206 (2014).

