While the 4 GeV data (and proposed 6 GeV extension) provide additional
information on the structure function at large ![]() , the main focus is the
study of the momentum distribution of nucleons in nuclei, and in particular
the nature of the high momentum components. The 4 GeV data from E89-008 showed
, the main focus is the
study of the momentum distribution of nucleons in nuclei, and in particular
the nature of the high momentum components. The 4 GeV data from E89-008 showed
![]() -scaling, with large
-scaling, with large ![]() -dependent scaling violations from final state
interactions below
-dependent scaling violations from final state
interactions below ![]() GeV
GeV![]() (Fig. 3).
Fig. 6 shows
(Fig. 3).
Fig. 6 shows ![]() for deuterium from the 4 GeV run. While the
data in the scaling region (
for deuterium from the 4 GeV run. While the
data in the scaling region (![]() GeV
GeV![]() ) is limited at large
) is limited at large ![]() , a clear
approach to scaling is observed. The solid line is a fit to
, a clear
approach to scaling is observed. The solid line is a fit to ![]() of the form
suggested in Ref. [34]:
of the form
suggested in Ref. [34]:
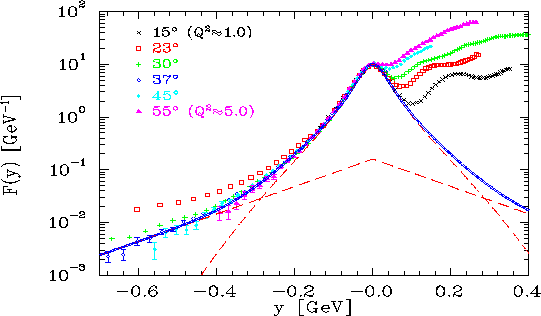 |
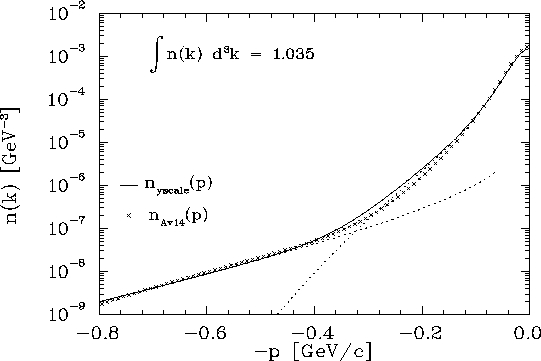
Fig. 7 shows the momentum distribution as extracted (using
Eq. 3) from the fit to ![]() , along with a calculation using the
Argonne v14 NN potential. The agreement is quite good, even at extremely large
values of
, along with a calculation using the
Argonne v14 NN potential. The agreement is quite good, even at extremely large
values of ![]() where
where ![]() is 4 to 5 orders of magnitude below the peak value.
In particular, the slope as well as the normalization on the tail agree quite
well with the calculated momentum distribution. The agreement in the tail
region, which is dominated by the short range interaction of the nucleons, seems
to indicate that the weakly
is 4 to 5 orders of magnitude below the peak value.
In particular, the slope as well as the normalization on the tail agree quite
well with the calculated momentum distribution. The agreement in the tail
region, which is dominated by the short range interaction of the nucleons, seems
to indicate that the weakly ![]() -dependent final state interactions suggested
for correlated nucleons do not make a large contribution to the scaling
function. The 4 GeV data are of limited quality at the large values of
-dependent final state interactions suggested
for correlated nucleons do not make a large contribution to the scaling
function. The 4 GeV data are of limited quality at the large values of ![]() ,
making it difficult to extract a precise shape and normalization for this tail.
Better data on deuterium, with the extended
,
making it difficult to extract a precise shape and normalization for this tail.
Better data on deuterium, with the extended ![]() range possible at higher
energy, should allow us to extract more detailed information about the tail of
the momentum distribution than we can get from the simple fit used in this
analysis. A precise measurement of this region will also allow us to set
significant limits on deviations from the momentum distribution due to possible
weakly
range possible at higher
energy, should allow us to extract more detailed information about the tail of
the momentum distribution than we can get from the simple fit used in this
analysis. A precise measurement of this region will also allow us to set
significant limits on deviations from the momentum distribution due to possible
weakly ![]() -dependent final state interactions of these strongly interacting
(small separation) nucleons.
-dependent final state interactions of these strongly interacting
(small separation) nucleons.
 |
While the ![]() -scaling analysis of the deuterium data yields results consistent
with exact calculations of the momentum distribution, this is not the case for
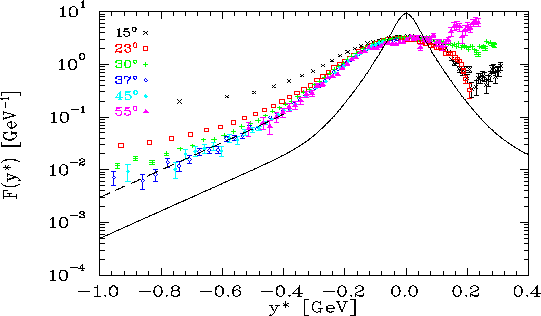
the heavy nuclei. Fig. 2 shows
-scaling analysis of the deuterium data yields results consistent
with exact calculations of the momentum distribution, this is not the case for
the heavy nuclei. Fig. 2 shows ![]() for iron. While the
data show
for iron. While the
data show ![]() -scaling, the falloff at large
-scaling, the falloff at large ![]() indicates that the high
momentum components (
indicates that the high
momentum components (
![]() GeV/c) are much smaller for iron than for
deuterium, even though one would expect more high momentum nucleons in the
denser nuclei. This is also true for the SLAC
GeV/c) are much smaller for iron than for
deuterium, even though one would expect more high momentum nucleons in the
denser nuclei. This is also true for the SLAC ![]() He data, where the extracted
He data, where the extracted
![]() is not consistent with calculations of the
is not consistent with calculations of the ![]() He momentum distribution.
In addition, the integral of
He momentum distribution.
In addition, the integral of ![]() should be unity in the
should be unity in the ![]() -scaling picture,
but the normalization of the scaling function for heavy nuclei are
-scaling picture,
but the normalization of the scaling function for heavy nuclei are ![]() 20-30%
low. For the
20-30%
low. For the ![]() He data, the normalization of the scaling function has been
analyzed in terms of ``swelling'' of the nucleon in the nucleus, and has been
used to set upper limit on medium modifications to the nucleon form
factors [35]. Extending such an analysis to heavier nuclei, where the
normalization is even lower, would lead to a prediction of greater modifications
to the nucleon radius. It is not clear that this kind of analysis gives
meaningful limits on nucleon medium modifications if there is a more fundamental
problem in the relation between
He data, the normalization of the scaling function has been
analyzed in terms of ``swelling'' of the nucleon in the nucleus, and has been
used to set upper limit on medium modifications to the nucleon form
factors [35]. Extending such an analysis to heavier nuclei, where the
normalization is even lower, would lead to a prediction of greater modifications
to the nucleon radius. It is not clear that this kind of analysis gives
meaningful limits on nucleon medium modifications if there is a more fundamental
problem in the relation between ![]() and
and ![]() . Nonetheless, tests of medium
modifications from
. Nonetheless, tests of medium
modifications from ![]() -scaling analyses has been used to set limits on nucleon
swelling used to explain the EMC effect.
-scaling analyses has been used to set limits on nucleon
swelling used to explain the EMC effect.
The rapid falloff of the scaling function at large values of ![]() indicates
that there is a failure of some kind in the PWIA scaling analysis. The breakdown
for
indicates
that there is a failure of some kind in the PWIA scaling analysis. The breakdown
for ![]() nuclei comes from the assumption that the residual nucleus remains
in an unexcited state (Eq. 2). The NN correlations responsible for
much of of the high momentum components are neglected. Clearly the
nuclei comes from the assumption that the residual nucleus remains
in an unexcited state (Eq. 2). The NN correlations responsible for
much of of the high momentum components are neglected. Clearly the ![]() spectator nucleus will not be in an unexcited state if one of a pair of very
high momentum nucleons is suddenly removed. The scaling violations arising from
the assumption of an unexcited final state have been treated in two ways. In
some cases, a correction to the scaling function is calculated, and the scaling
function that is related directly to the momentum distribution is
spectator nucleus will not be in an unexcited state if one of a pair of very
high momentum nucleons is suddenly removed. The scaling violations arising from
the assumption of an unexcited final state have been treated in two ways. In
some cases, a correction to the scaling function is calculated, and the scaling
function that is related directly to the momentum distribution is
![]() , where
, where ![]() is the measured scaling function, and
is the measured scaling function, and ![]() is the
calculated binding correction. A more direct way to take the excitation of the
residual nucleus into account is to determine an excitation energy for the
residual system based on a modified picture of the interaction that includes the
correlations. Rather than having the momentum of the struck nucleon balanced by
the residual nucleus, it's momentum is balanced by a single nucleon, and
the residual
is the
calculated binding correction. A more direct way to take the excitation of the
residual nucleus into account is to determine an excitation energy for the
residual system based on a modified picture of the interaction that includes the
correlations. Rather than having the momentum of the struck nucleon balanced by
the residual nucleus, it's momentum is balanced by a single nucleon, and
the residual ![]() nucleus is at rest [36], or has a small recoil
momentum [34]. We have performed an analysis of this kind using a
modified definition of
nucleus is at rest [36], or has a small recoil
momentum [34]. We have performed an analysis of this kind using a
modified definition of ![]() , based on a simple three-body breakup of the nucleus,
where the nucleon struck is assumed to be one of a correlated pair that is
moving in the nucleus with a momentum (along the
, based on a simple three-body breakup of the nucleus,
where the nucleon struck is assumed to be one of a correlated pair that is
moving in the nucleus with a momentum (along the ![]() vector) equal to
vector) equal to ![]() .
In the limit where
.
In the limit where ![]() is much larger than the momenta of the nucleons (and thus
the transverse component of these momenta can be ignored), energy conservation
gives:
is much larger than the momenta of the nucleons (and thus
the transverse component of these momenta can be ignored), energy conservation
gives:
The scaling variable in this case is
![]() , the total initial
momentum of the struck nucleon. Note that for the deuteron, there is no
, the total initial
momentum of the struck nucleon. Note that for the deuteron, there is no ![]() residual nucleus and thus no
residual nucleus and thus no ![]() , so
, so ![]() is just the usual scaling
variable
is just the usual scaling
variable ![]() . Eq. 5 cannot directly be solved for
. Eq. 5 cannot directly be solved for ![]() without a relation between
without a relation between ![]() and
and ![]() . This is obtained by convolving
the center of mass motion of the quasi-deuteron with the relative momentum of
the nucleons in the pair (taken to be identical to the real deuteron momentum
distribution). This allows us to determine the average
. This is obtained by convolving
the center of mass motion of the quasi-deuteron with the relative momentum of
the nucleons in the pair (taken to be identical to the real deuteron momentum
distribution). This allows us to determine the average ![]() for a given
value of the initial nucleon momentum.
for a given
value of the initial nucleon momentum.
Fig. 8 shows the modified scaling function, ![]() , along with
a fit to to
, along with
a fit to to ![]() (dashed line). In the figure we have made a subtraction
of the inelastic contribution which is significant for
(dashed line). In the figure we have made a subtraction
of the inelastic contribution which is significant for
![]() . Note
that the behavior at very large negative
. Note
that the behavior at very large negative ![]() is very different from the
behavior in Fig. 2, and that the data is sensitive to larger values
of initial nucleon momentum that one would assume based on the usual
is very different from the
behavior in Fig. 2, and that the data is sensitive to larger values
of initial nucleon momentum that one would assume based on the usual ![]() -scaling
function (probing nuclei with initial momenta of more than 1 GeV/c). However, at
these very large
-scaling
function (probing nuclei with initial momenta of more than 1 GeV/c). However, at
these very large ![]() values, the uncertainties in the 4 GeV data are large,
and the data just barely reach
values, the uncertainties in the 4 GeV data are large,
and the data just barely reach ![]() values where scaling appears to have set
in. With data at higher
values where scaling appears to have set
in. With data at higher ![]() values in this large
values in this large ![]() region, we should be
able to map out the high momentum tail of the nucleon momentum distribution.
Also notice that the shape of the scaling function at large negative
region, we should be
able to map out the high momentum tail of the nucleon momentum distribution.
Also notice that the shape of the scaling function at large negative ![]() values is the same as for the deuteron, but is roughly 6 times larger (dashed
line), indicating that the high momentum components appear to be well described
by two nucleon correlations. This is the same behavior one sees when examining
the ratio of the structure functions in the region of
values is the same as for the deuteron, but is roughly 6 times larger (dashed
line), indicating that the high momentum components appear to be well described
by two nucleon correlations. This is the same behavior one sees when examining
the ratio of the structure functions in the region of
![]() , where
the ratio is flat and roughly 5 to 6 times higher in heavy
nuclei [37,15]. With better data for deuterium at large
initial nucleon momenta, this comparison could be much more quantitative, and we
could look for signs of high momentum components beyond the two nucleon
correlations. In addition, data on
, where
the ratio is flat and roughly 5 to 6 times higher in heavy
nuclei [37,15]. With better data for deuterium at large
initial nucleon momenta, this comparison could be much more quantitative, and we
could look for signs of high momentum components beyond the two nucleon
correlations. In addition, data on ![]() He will allow for direct comparison of
high momentum nucleons (
He will allow for direct comparison of
high momentum nucleons (![]() ) in heavy nuclei to three nucleon correlations.
) in heavy nuclei to three nucleon correlations.
 |
Of course one does not have to rely on a non-relativistic, impulse approximation scaling analysis to study the momentum distribution and the high momentum components. One can do a complete, relativistic calculation of the cross section, using the full spectral function and including final state interactions. One can also use a relativistic scaling analysis which gives a different scaling variable and connects to light-cone momentum distributions, as suggested in Ref. [6]. The scaling analysis discussed here is intended to give a feel for the coverage and significance of the data, but also to show the success of this simple scaling analysis. This success gives us confidence in the underlying assumptions.
The question of the nature of the short range correlations can also be
examined without relying on a ![]() -scaling analysis, by directly examining the
structure functions. Fig. 9 shows a calculation of the structure
function per nucleon for iron, including just two nucleon correlations (solid
line - from [38]), and including multinucleon correlations (dotted
line - from [13]). The current data clearly indicate that the effect
of multinucleon correlations is significantly smaller than estimated in the
calculation. The calculation for the two nucleon SRC contributions does not
include corrections for the EMC effect, but such a calculation should be
available very soon [39]. The inclusion of the EMC effect will
lower the calculations somewhat, making it difficult to use this data to set a
strong upper limit on multinucleon components. An extension to 6 GeV will allow
us to reach
-scaling analysis, by directly examining the
structure functions. Fig. 9 shows a calculation of the structure
function per nucleon for iron, including just two nucleon correlations (solid
line - from [38]), and including multinucleon correlations (dotted
line - from [13]). The current data clearly indicate that the effect
of multinucleon correlations is significantly smaller than estimated in the
calculation. The calculation for the two nucleon SRC contributions does not
include corrections for the EMC effect, but such a calculation should be
available very soon [39]. The inclusion of the EMC effect will
lower the calculations somewhat, making it difficult to use this data to set a
strong upper limit on multinucleon components. An extension to 6 GeV will allow
us to reach ![]() GeV
GeV![]() at
at ![]() , where the calculation predicts very
large contribution from multinucleon correlations. In addition, with data on
, where the calculation predicts very
large contribution from multinucleon correlations. In addition, with data on
![]() H,
H, ![]() He, and
He, and ![]() He, it should be possible to disentangle the EMC effect
from 3N correlations [39,40]. This will allow us to
either obtain a clear signal of multinucleon correlations, or set significant
limits on their contributions. We can also directly compare the structure
function for heavy nuclei to few body nuclei in the region where the structure
function is dominated by SRCs. By comparing heavy nuclei to deuterium, we can
look for deviations from the two nucleon SRCs, and by comparing to
He, it should be possible to disentangle the EMC effect
from 3N correlations [39,40]. This will allow us to
either obtain a clear signal of multinucleon correlations, or set significant
limits on their contributions. We can also directly compare the structure
function for heavy nuclei to few body nuclei in the region where the structure
function is dominated by SRCs. By comparing heavy nuclei to deuterium, we can
look for deviations from the two nucleon SRCs, and by comparing to ![]() He where
the two nucleon correlations are small for
He where
the two nucleon correlations are small for ![]() , we can look for signatures
of three nucleon correlations. This type of comparison is more direct than
comparisons of the extracted momentum distribution from a scaling analysis. In
addition, if there are significant final state interactions between correlated
nucleons at large
, we can look for signatures
of three nucleon correlations. This type of comparison is more direct than
comparisons of the extracted momentum distribution from a scaling analysis. In
addition, if there are significant final state interactions between correlated
nucleons at large ![]() values, these should cancel to first order in these
ratios.
values, these should cancel to first order in these
ratios.
 |